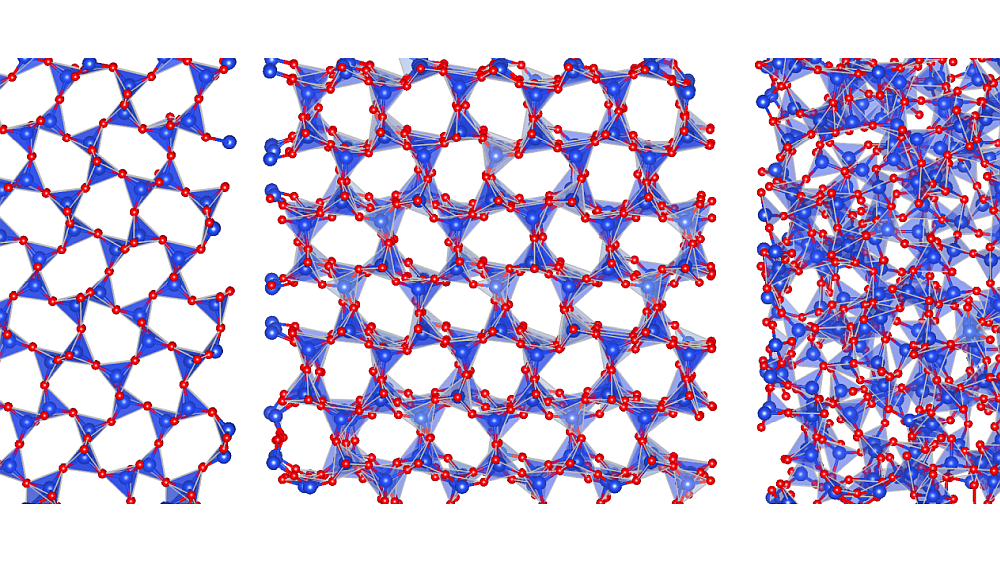
[Image above] Ordered and disordered materials transport heat quite differently based on their contrasting atomic structures. A new theory can describe thermal transport in both. Credit: Raquelaa54, Wikimedia (CC BY 4.0)
When I conducted glass research as an undergraduate, I learned to keep an eye on first-years when they asked to use one of my alumina crucibles to melt their glass. The majority of students used platinum crucibles for research, and alumina crucibles were used only when there were concerns about the starting powders reacting with platinum.
If I did not watch the first-years carefully, they would pull the alumina crucible from the oven, wait the few minutes necessary for a platinum crucible to cool, and then try to pick it up—and their yelps of surprise let me know they learned the hard way that ceramic cools a lot slower than metal.
Many adults intuitively know which situations cause an object to become hot based on “experiments” they conducted as children (remember touching the hot stove your parents warned you about?).
This knowledge based on observational factors like color change and phase transitions serves well as a basis for identifying heat, but knowing when an object has cooled is another matter. As the first-years showed, they knew an object pulled from the oven was hot—they did not know metals and ceramics release that heat at different rates.
This discrepancy between knowing what causes an object to become hot and knowing when it will cool comes down to knowing how an object conducts heat. And in the case of ordered and disordered materials such as crystals and glass, these materials exhibit fundamentally different heat conduction mechanisms.
In crystals, the regular arrangement of atoms means that heat is conducted quickly by propagation of vibrational waves. Physicist Rudolf Peierls laid the foundation for describing crystal heat transfer in 1929, and his semi-classical theory—based on the assumption that phonons obey the Boltzmann transport equation (BTE)—is still used today.
In contrast, the disordered arrangement of atoms in glass means heat is transferred much more slowly by random hopping of vibrations. Scientists Philip Allen (Stony Brook University) and Joseph Feldman (United States Naval Research Laboratory) developed this harmonic theory of thermal transport in 1989.
Until now, these two theories for describing thermal transport in crystals and glass have stayed just as that—two separate theories. But based on new research by Swiss and Italian researchers, these two theories may be more similar than previously believed.
Michele Simoncelli (PhD student) and Nicola Marzari (professor; director of NCCR MARVEL) at Swiss Federal Institute of Technology Lausanne–EPFL, and professor Francesco Mauri at the University of Rome Sapienza derived a general formula of thermal transport that describes equally well both ordered and disordered materials, as well as everything in between. Depending on what limits they place on the formula, it reduces to either the BTE for simple crystals or the Allen-Feldman formulation for harmonic glasses.
The key to developing their general formula was accounting for a fundamental component of heat propagation that Peierls discarded—quantum tunneling. “[Phonon wave packets] are not only allowed to propagate particle-like in space but also to tunnel, wave-like, from one branch to another,” the researchers explain in their paper. While such tunneling contributions are negligible in perfect crystals, the tunnels become more relevant as system disorder increases. In a glass, these tunnels result in the Allen-Feldman formalism.
To account for quantum tunneling, the researchers derived their general transport equation from the Wigner phase space formulation of quantum mechanics. They then showed how this general equation can be reduced to both the BTE and Allen-Feldman formulations.
More than uniting the BTE and Allen-Feldman formulations, the largest benefit of the general theory is the ability to calculate heat transport for intermediate materials that are both crystal-like and glass-like—specifically, thermoelectrics.
Thermoelectrics are materials that can convert temperature differences to electric voltage and vice versa. Thermoelectric materials hold enormous potential in energy applications to generate electricity from “waste” heat in industrial processes.
Materials that are both crystal-like and glass-like make for good thermoelectrics, but until now, treating these materials strictly in terms of either crystal or glass based on available heat transport equations resulted in large errors.
Using the new general theory, though, can improve these calculations. As the NCCR MARVEL press release notes, “The new understanding outlined in the paper and more accurate estimates of thermal conductivity, along with data on the electrical conductivity, will allow scientists to calculate the ‘figure of merit’ of thermoelectrics, and provide an estimate of their efficiency.”
The paper, published in Nature Physics, is “Unified theory of thermal transport in crystals and glasses” (DOI: 10.1038/s41567-019-0520-x).